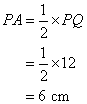CIRCLES
Definition of Terms
Central Angle
The Formula for the Length of an Arc
Derive the formula for the length of an arc
Circumference of Circle = PI x diameter = 2 PI x radius where PI =𝝅= 3.141592…
The Central Angle
Calculate the central angle
A central angle is an angle formed by two intersecting radii such that its vertex is at thecenter of the circle.
<AOB is a central angle. Its intercepted arc is the minor arc from A to B.
The Concept of Radian Measure
Explain the concept of radian measure
Radians
are the standard mathematical way to measure angles. One radian is
equal to the angle created by taking the radius of a circle and
stretching it along the edge of the circle.
are the standard mathematical way to measure angles. One radian is
equal to the angle created by taking the radius of a circle and
stretching it along the edge of the circle.
The
radian is a pure mathematical measurement and therefore is preferred by
mathematicians over degree measures. For use in everyday work, the
degree is easier to work with, but for purely mathematical pursuits, the
radian gives better results. You probably will never see radian
measures used in construction or surveying, but it is a common unit in
mathematics and physics.
radian is a pure mathematical measurement and therefore is preferred by
mathematicians over degree measures. For use in everyday work, the
degree is easier to work with, but for purely mathematical pursuits, the
radian gives better results. You probably will never see radian
measures used in construction or surveying, but it is a common unit in
mathematics and physics.
Radians to Degree and Vice Versa
Convert radians to degree and vice versa
The
unit used to describe the measurement of an angle that is most familiar
is thedegree. To convert radians to degrees or degrees to radians, the
following relationship can be used.
unit used to describe the measurement of an angle that is most familiar
is thedegree. To convert radians to degrees or degrees to radians, the
following relationship can be used.
angle in degrees = angle in radians * (180/pi)
So, 180 degrees = pi radians
Example 1
Convert 45 degrees to radians
Solution
45 = 57.32*radians
radians = 45/57.32
radians = 0.785
Most
often when writing degree measure in radians, pi is not calculated in,
so for this problem, the more accurate answer would beradians = 45
pi/180 = pi/4
often when writing degree measure in radians, pi is not calculated in,
so for this problem, the more accurate answer would beradians = 45
pi/180 = pi/4
Example 2
Convert pi/3 radians to degree
degrees = (pi/3) * (180/pi)
degrees = 180/3 = 60°
Angles Properties
Circle Theorems of Inscribed Angles
Prove circle theorems of inscribed angles
Aninscribedangle
is formed when two secant lines intersect on a circle. It can also be
formed using a secant line and a tangent line intersecting on a circle. Acentral angle,
on the other hand, is an angle whose vertex is the center of the circle
and whose sides pass through a pair of points on the circle, therefore
subtending an arc.In this post, we explore the relationship between
inscribed angles and central angles having the same subtended arc. The
angle of the subtended arc is the same as the measure of the central
angle (by definition).
is formed when two secant lines intersect on a circle. It can also be
formed using a secant line and a tangent line intersecting on a circle. Acentral angle,
on the other hand, is an angle whose vertex is the center of the circle
and whose sides pass through a pair of points on the circle, therefore
subtending an arc.In this post, we explore the relationship between
inscribed angles and central angles having the same subtended arc. The
angle of the subtended arc is the same as the measure of the central
angle (by definition).
In
the first circle,is a central angle subtended by arc. Angleis an
inscribed angle subtended by arc. In the second circle,is an inscribed
angle andis a central angle. Both angles are subtending arc.
the first circle,is a central angle subtended by arc. Angleis an
inscribed angle subtended by arc. In the second circle,is an inscribed
angle andis a central angle. Both angles are subtending arc.
What
can you say about the two angles subtending the same arc? Draw several
cases of central angles and inscribed angles subtending the same arc and
measure them. Use a dynamic geometry software if necessary. Are your
observations the same?
can you say about the two angles subtending the same arc? Draw several
cases of central angles and inscribed angles subtending the same arc and
measure them. Use a dynamic geometry software if necessary. Are your
observations the same?
In
the discussion below, we prove one of the three cases of the
relationship between a central angle and an inscribed angle subtending
the same arc.
the discussion below, we prove one of the three cases of the
relationship between a central angle and an inscribed angle subtending
the same arc.
Theorem
The
measure of an angle inscribed in a circle is half the measure of the
arc it intercepts. Note that this is equivalent to the measure of the
inscribed angle is half the measure of the central angle if they
intercept the same arc.
measure of an angle inscribed in a circle is half the measure of the
arc it intercepts. Note that this is equivalent to the measure of the
inscribed angle is half the measure of the central angle if they
intercept the same arc.
Proof
Letbe
an inscribed angle andbe a central angle both subtending arcas shown in
the figure. Draw line. This forms two isosceles trianglesandsince two
of their sides are radii of the circle.
an inscribed angle andbe a central angle both subtending arcas shown in
the figure. Draw line. This forms two isosceles trianglesandsince two
of their sides are radii of the circle.
In
triangle, if we let the measure ofbe, then angleis also. By theexterior
angle theorem, the measure of angle. This is also similar to triangle.
If we let angle, it follows thatis equal to 2y. In effect, the measure of the inscribed angleand the measure of central anglewhich is what we want to prove.
triangle, if we let the measure ofbe, then angleis also. By theexterior
angle theorem, the measure of angle. This is also similar to triangle.
If we let angle, it follows thatis equal to 2y. In effect, the measure of the inscribed angleand the measure of central anglewhich is what we want to prove.
The Circle Theorems in Solving Related Problems
Apply the circle theorems in solving related problems
Example 3
An arc subtends an angle of 200 at the center of the circle of radius25cm.Find the length of this arc.
Solution
r =25cm, 𝜽=20°
The length of the arc is 8.73cm.
Example 4
An arc of length 5cm subtends 50° at the center of the circle, what is theradius of the circle?
l=5cm, 𝜽=50°, r=?
Chord Properties of a Circle
Chord Properties of a Circle
Identify chord properties of a circle
Imagine
that you are on one side of a perfectly circular lake and looking
across to a fishing pier on the other side. The chord is the line going
across the circle from point A (you) to point B (the fishing pier). The
circle outlining the lake’s perimeter is called thecircumference. Achord
of a circleis a line that connects two points on a circle’s
circumference.
that you are on one side of a perfectly circular lake and looking
across to a fishing pier on the other side. The chord is the line going
across the circle from point A (you) to point B (the fishing pier). The
circle outlining the lake’s perimeter is called thecircumference. Achord
of a circleis a line that connects two points on a circle’s
circumference.
To
illustrate further, let’s look at several points of reference on the
same circular lake from before. If each point of reference (i.e. duck
feeding area, picnic tables, you, water fountain, and fishing pier) were
directly on this lake’s circumference, then each line connecting a
point to another point on the circle would be chords.
illustrate further, let’s look at several points of reference on the
same circular lake from before. If each point of reference (i.e. duck
feeding area, picnic tables, you, water fountain, and fishing pier) were
directly on this lake’s circumference, then each line connecting a
point to another point on the circle would be chords.
- The line between the fishing pier and you is now chord AC
- The line between the water fountain and duck feeding area is now chord BE
- The line between you and the picnic tables is chord CD
If
we had a chord that went directly through the center of a circle, it
would be called adiameter. If we had a line that did not stop at the
circle’s circumference and instead extended into infinity, it would no
longer be a chord; it would be called asecant.
we had a chord that went directly through the center of a circle, it
would be called adiameter. If we had a line that did not stop at the
circle’s circumference and instead extended into infinity, it would no
longer be a chord; it would be called asecant.
The Theorem on the Perpendicular Bisector to a Chord
Prove the theorem on the perpendicular bisector to a chord.
Proof of Theorem
The Theorem on Parallel Chords
Prove the theorem on parallel chords
Parallel chords in the same circle always cut congruent arcs. Parallel chords intercept congruent arcs.
- Construct a diameter perpendicular to the parallel chords.
- What does this diameter do to each chord? The diameter bisects each chord.
- Reflect across the diameter (or fold on the diameter). What happens to the endpoints?The
reflection takes the endpoints on one side to the endpoints on the
other side. It, therefore, takes arc to arc. Distances from the center
are preserved. - What have we proven? Arcs between parallel chords are congruent.
The Theorems on Chords in Solving Related Problems
Apply the theorems on chords in solving related problems
Example 5
The figure is a circle with centreO. GivenPQ= 12 cm. Find the length ofPA.
Solution:
The radiusOBis perpendicular toPQ. So,OBis a perpendicular bisector ofPQ.
Example 6
The figure is a circle with centreOand diameter 10 cm.PQ= 1 cm. Find the length ofRS.
Solution:
A Tangent to a Circle
Describe a tangent to a circle
Tangent
is a line which touches a circle. The point where the line touches the
circle is called the point of contact. A tangent is perpendicular to the
radius at the point of contact.
is a line which touches a circle. The point where the line touches the
circle is called the point of contact. A tangent is perpendicular to the
radius at the point of contact.
Tangent Properties of a Circle
Identify tangent properties of a circle
A
tangent to a circle is perpendicular to the radius at the point of
tangency. A common tangent is a line that is a tangent to each of two
circles. A common external tangent does not intersect the segment that
joins the centers of the circles. A common internal tangent intersects
the segment that joins the centers of the circles.
tangent to a circle is perpendicular to the radius at the point of
tangency. A common tangent is a line that is a tangent to each of two
circles. A common external tangent does not intersect the segment that
joins the centers of the circles. A common internal tangent intersects
the segment that joins the centers of the circles.
Tangent Theorems
Prove tangent theorems
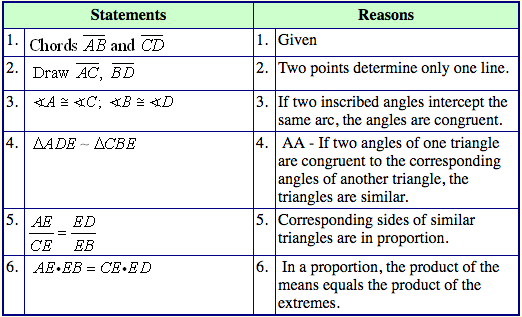
Theorem 1
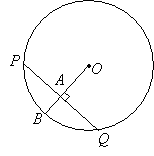
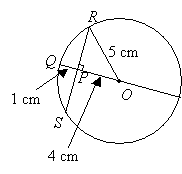
If
two chords intersect in a circle, the product of the lengths of the
segments of one chord equal the product of the segments of the other.
two chords intersect in a circle, the product of the lengths of the
segments of one chord equal the product of the segments of the other.
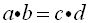
Intersecting Chords Rule: (segment piece)×(segment piece) =(segment piece)×(segment piece)
Theorem Proof:
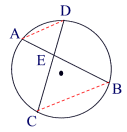
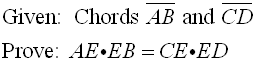
Theorem 2:
If
two secant segments are drawn to a circle from the same external point,
the product of the length of one secant segment and its external part
is equal to the product of the length of the other secant segment and
its external part.
two secant segments are drawn to a circle from the same external point,
the product of the length of one secant segment and its external part
is equal to the product of the length of the other secant segment and
its external part.
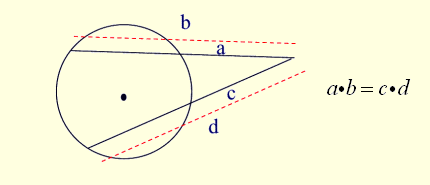
Secant-Secant Rule: (whole secant)×(external part) =(whole secant)×(external part)
Theorem 3:
If
a secant segment and tangent segment are drawn to a circle from the
same external point, the product of the length of the secant segment and
its external part equals the square of the length of the tangent
segment.
a secant segment and tangent segment are drawn to a circle from the
same external point, the product of the length of the secant segment and
its external part equals the square of the length of the tangent
segment.
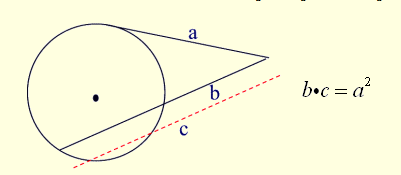
Secant-Tangent Rule:(whole secant)×(external part) =(tangent)2
Theorems Relating to Tangent to a Circle in Solving Problems
Apply theorems relating to tangent to a circle in solving problems
Example 7
Two
common tangents to a circle form a minor arc with a central angle of
140 degrees. Find the angle formed between the tangents.
common tangents to a circle form a minor arc with a central angle of
140 degrees. Find the angle formed between the tangents.
Solution
Two tangents and two radii form a figure with 360°. If y is the angle formed between the tangents then y + 2(90) + 140° = 360°
y = 40°.
The angle formed between tangents is 40 degrees.
