GEOMETRIC AND TRANSFORMATIONS
Reflection
The Characteristics of Reflection in a Plane
Describe the characteristics of reflection in a plane
A transformation in a plane is a mapping which moves an object from one position to another within the plane. Think of a book being taken from one comer of a table to another comer. Figures on a plane of paper can also be shifted to a new position by a transformation. The new postion after a transformation is called the image. Examples of transformations are reflection, rotation, enlargement and translation.
Different Reflections by Drawings
Represent different reflections by drawings
A reflection is a transformation which reflects all points of a plane in a line called the mirror-line. The image in a mirror is as far behind the mirror as the object is in front of the mirror
Characteristics of Reflection
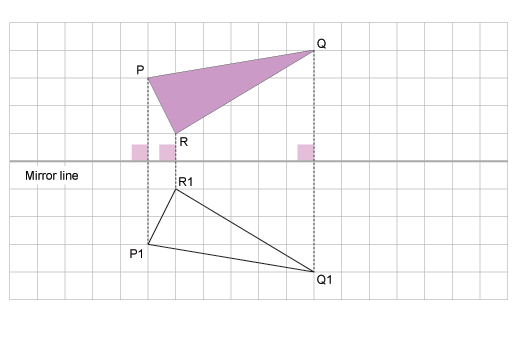
In the diagram, APQR is mapped onto ΔP’Q’R’ under a reflection in the line AB. If the paper is folded along the line AB, ΔPQR will fall in exactly onto ΔPQR. The line AB is the mirror-line. which is the perpendicular bisector of PP’, QQ’ and ΔPQR and ΔP’Q’R are congruent.
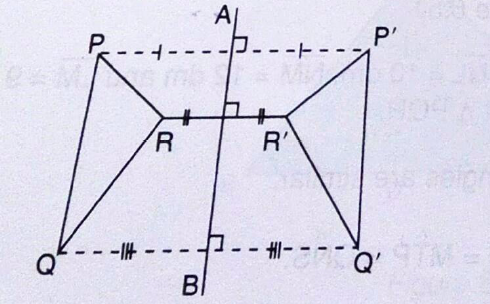
Some characteristics observed under reflection are:
- PP’ is perpendicular to AB, RR’ is perpendicular to AB and QQ is perpendicular to AB.
- The image of any point on the Q’ mirror line is the point itself.
- PP’ is parallel to RR’ and QQ’
Reflection in the Line y = x
The
line y = x makes an angle 45° with the x and y axes. It is the line of
symmetry for the angle YOX formed by the two axes. By using the
isosceles triangle properties, reflection of the point (1, 0) in the
line y = x will be (0, 1).
line y = x makes an angle 45° with the x and y axes. It is the line of
symmetry for the angle YOX formed by the two axes. By using the
isosceles triangle properties, reflection of the point (1, 0) in the
line y = x will be (0, 1).
The
reflection of (0,2) in the liney = x will be (2,0). You notice that the
co-ordinates are exchanging positions. Generally, the reflection of the
point (a,b) in the line y = x is (b,a).
reflection of (0,2) in the liney = x will be (2,0). You notice that the
co-ordinates are exchanging positions. Generally, the reflection of the
point (a,b) in the line y = x is (b,a).
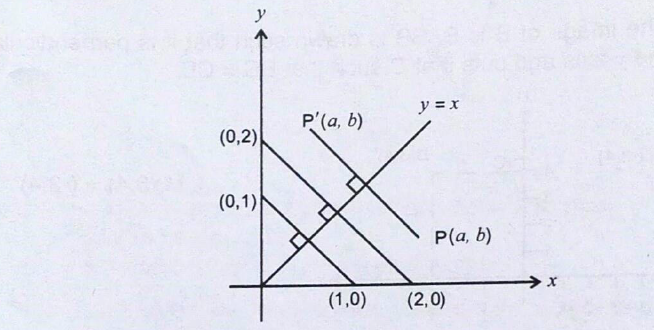
The reflection of the point B(c,d) in the line y = -x is B’ (-d, -c)
Exercise 1
- Find the image of the point D(4,2) under a reflection in the x-axis.
- Find the image of the point P(-2,5) under a reflection in the x-axis.
- Point Q(-4,3) is reflected in the y-axis. Find the coordinates of its image.
- Point R(6,-5) is reflected in the y-axis. Find the co-ordinates of its image.
- Reflect the point (1 ,2) in the line y = -x.
- Reflect the point (5,3) in the line y = x.
- Find the image of the point (1 ,2) after a reflection in the line y=x followed by another reflection in the line y = -x.
- Find
the image of the point P(-2,1) in the line y = -x followed by another
reflection in the line x = 0 ketch the positions of the image P and the
point P, indicating clearly the lines involved. - Find the co-ordinates of the image of the point A(5,2) under a reflection in the line y = 0.
- Find the coordinates of the image of the point under a reflection in the line x = 0.
- The co-ordinates of the image of a point R reflected in the x axis is R(2, -9). Find the coordinates of R.
Rotations
Characteristics of a Rotation on a Plane
Describe characteristics of a rotation on a plane
Rotation
is a transformation which rotates all points on a plane about a fixed
point known as the centre of rotation through a given angle in a
clockwise or anticlockwise direction
is a transformation which rotates all points on a plane about a fixed
point known as the centre of rotation through a given angle in a
clockwise or anticlockwise direction
In order to describe a rotation, we give:
- the centre of rotation,
- the angle of rotation, and
- the direction of rotation
Example 1
Copy the figure T and rotate 180° about the origin.
Different Rotation on a Plane by Drawings
Represent different rotation on a plane by drawings
When
you turn a ruler at its end corner through an angle 0° it make a
rotation. A rotation is transformation which moves a point through a
given angle about a fixed point.
you turn a ruler at its end corner through an angle 0° it make a
rotation. A rotation is transformation which moves a point through a
given angle about a fixed point.
A
rotation is a transformation that turns a figure about a fixed point
called a Centre of Rotation.You can rotate the figure as much as 360
degrees. The transformation of Rotation is usually denoted by R. The
symbol R› means that an object is rotated through an angle 9. In the xy
plane, when is measured in the clockwise direction it is negative and
when it is measured in the anticlockwise direction it is positive.
rotation is a transformation that turns a figure about a fixed point
called a Centre of Rotation.You can rotate the figure as much as 360
degrees. The transformation of Rotation is usually denoted by R. The
symbol R› means that an object is rotated through an angle 9. In the xy
plane, when is measured in the clockwise direction it is negative and
when it is measured in the anticlockwise direction it is positive.
P is on the x-axis
After
a rotation through 90° about the origin it will be on the y-axis. Since
P is 1 from O, P’ is 1 from O, the coordinates of P are P'(O,I). Hence R90°( 1, O) = (O, 1)
a rotation through 90° about the origin it will be on the y-axis. Since
P is 1 from O, P’ is 1 from O, the coordinates of P are P'(O,I). Hence R90°( 1, O) = (O, 1)
Exercise 2
- Find the image of the point (1, 2) under a rotation through 180° anti-clockwise about the origin.
- Find the rotation of the point (6, 0) under a rotation through 90° Clockwise about the origin.
- Find the rotation of the point (-2, 1) under a rotation through 270° clockwise about the origin.
- Point Q(5, -4) is rotated through 270° in the clockwise direction. Find the coordinates of its image.
- Find the image of (1, 2) after a rotation of -90°
- Find the image of (-3, 5) after a rotation of -180°
- Find the image of (-5, 0) after a rotation of -180°
- Find the image of (-5, 0) after a rotation of 180° about the origin. Comment about the results of questions 7 and 8.
- The
vertices of triangle OAB are O(0,0), A(2,3) and B(2,1). The triangle is
rotated through 90° anti-clockwise about the origin. Find the
co-ordinates of its image. - The vertices of rectangle PQRS are
P(0,0), Q(3,0), R(2,3), S(0,2). The rectangle is rotated through 90°
clockwise about the origin. a) Find the co-ordinates of its image b)
draw the image.
Translation
Properties of Translations
State properties of translations
Translation is a transformation which moves all points, on a plane through the same direction
In
the diagram, ΔABC slidesΔA’B’C’ (A’ is read as A prime) is the
direction AA’. Note that AA’ are parallel and of equal length. We say
that ABC is mapped onto A’B’C’ by a translation.
the diagram, ΔABC slidesΔA’B’C’ (A’ is read as A prime) is the
direction AA’. Note that AA’ are parallel and of equal length. We say
that ABC is mapped onto A’B’C’ by a translation.
A
translation usually denoted by T. For example 1(1, 1) = (6, 1) means
that the point 1(1,1) has been moved to (6, 1) by a translation T. This
translation will move theorigin (0,0) to (5,0) and is written as T =
5/0.
translation usually denoted by T. For example 1(1, 1) = (6, 1) means
that the point 1(1,1) has been moved to (6, 1) by a translation T. This
translation will move theorigin (0,0) to (5,0) and is written as T =
5/0.
Translations Drawings
Represent translations drawings
Translation drawings
If
you cast a shadow of an object onto a plane surface, say a wall, by
using a light source, the shadow becomes bigger as the object moves
closer to the light source.
you cast a shadow of an object onto a plane surface, say a wall, by
using a light source, the shadow becomes bigger as the object moves
closer to the light source.
In
figure 7.40, AB casts a shadow CD. When AB moves to a new position and
is renamed QP (same size with AB) the shadow of QP becomes SR and it is
greater than CD.
figure 7.40, AB casts a shadow CD. When AB moves to a new position and
is renamed QP (same size with AB) the shadow of QP becomes SR and it is
greater than CD.
Enlargement
A Scale of Enlargement
Develop a scale of enlargement
Enlargement
is a transformation in which a figure is made larger (magnified) or
made smaller (diminished). A photograph may be enlarged or diminished to
suit a Certain purpose. Figures can be drawn to scale where actual
figures are diminished or enlarged. Enlarged shapes are geometrically
similar and have corresponding angles equal. The number that magnifies
or diminishes a figure is called the enlargement factor and is usually
denoted by k. If k is less than 1 the figure is diminished and if it is
greater that 1 the figure is enlarged k times.
is a transformation in which a figure is made larger (magnified) or
made smaller (diminished). A photograph may be enlarged or diminished to
suit a Certain purpose. Figures can be drawn to scale where actual
figures are diminished or enlarged. Enlarged shapes are geometrically
similar and have corresponding angles equal. The number that magnifies
or diminishes a figure is called the enlargement factor and is usually
denoted by k. If k is less than 1 the figure is diminished and if it is
greater that 1 the figure is enlarged k times.
In the case of closed figures, if the lengths enlarged by a factor of k then the area is enlarged by k2.
Scale
Similarity
can be used in enlarging of diminishing geometrical figures. For
example in maps, a large area of land is represented by a small area on
paper by a scale. Scale is a ratio between the measurement of a drawing
to the actual measurement. It is normally stated in the form of 1 : n,
for example, if a scale of a map is 1.20000, then 1 unit on the map
represents 20000 units on the ground.
can be used in enlarging of diminishing geometrical figures. For
example in maps, a large area of land is represented by a small area on
paper by a scale. Scale is a ratio between the measurement of a drawing
to the actual measurement. It is normally stated in the form of 1 : n,
for example, if a scale of a map is 1.20000, then 1 unit on the map
represents 20000 units on the ground.
In the figure, triangle ABC is a scale measurement of triangle PQR, where the scale is 1:2.
Scale is measurement of drawing: Actual Measurement, that is,
Scale = Measurement of drawing/Actual measurement
Exercise 3
Find the length of a drawing that represents:
- 15 km when the scale is 1:500000
- 45 km when the scale is 1 km to 900 m.
A building2 50 metres high is representedb y a line segment of length 5 cm. Finc the scale of the drawing.
A
triangular plot of land has sides 152 metres and 208 metres meeting at
an angle of 500. Find by scale drawing the distance from the middle
point of the longest side to the opposite corner
triangular plot of land has sides 152 metres and 208 metres meeting at
an angle of 500. Find by scale drawing the distance from the middle
point of the longest side to the opposite corner
Enlargement of a Given Figures
Construct enlargement of a given figures
Consider two similar rectangles shown in the figure below with a scale factor k.
If AB = a and AD = a, then PO = ak and PS bk
Area of ABCD = a× a = ab
Area of PORS = ak x bk = abk2
Area of PORS/Area of ABCD = abk2/ab = k2
Therefore, if two polygons have a scale factor k then the ratio of their areas is k2. This is also called the scale factor for the area.
Figures to Scale
Draw figures to scale
If we ‘enlarge’ a shape by a scale factor that is between -1 and 1, the image will besmallerthan the object
Example 2
Enlarge triangleABCwith a scale factor1/2, centred about the origin.
Solution
The scale factor is1/2, so:
OA’ =1/2OA
OB’ =1/2OB
OC’ =1/2OC
Since the centre is the origin, we can in this case multiply each coordinate by1/2to get the answers.
A = (2, 2), so A’ will be (1, 1).
B = (2, 6), so B’ will be (1, 3).
C = (4, 2), so C’ will be (2, 1).
Actual Distances Represented by a Scale Drawings
Find actual distances represented by a scale drawings
If
two polygons are similar and the ratio of their corresponding sides of
two similar polygons is 5:3, then the scale of enlargement is 5/3
two polygons are similar and the ratio of their corresponding sides of
two similar polygons is 5:3, then the scale of enlargement is 5/3
Exercise 4
- Two triangles are similar but not congruent. Is one the enlargement of the other?
- The length of a rectangle is twice the length of another rectangle. Is one necessarily an enlargement of the other? Explain.
- In the figure below BC DE, AB 5 cm, BD = 3cm and EF = 4 cm
- State which triangle is an enlargement of ΔABC
- Calculate the scale factor of the enlargement.
Combined Transformations
Draw combined transformations
Combined
Transformation means that two or more transformations will be Performed
on one object. For instance you could perform a reflection and then a
translation on the same point
Transformation means that two or more transformations will be Performed
on one object. For instance you could perform a reflection and then a
translation on the same point
Example 3
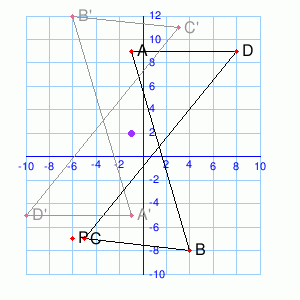
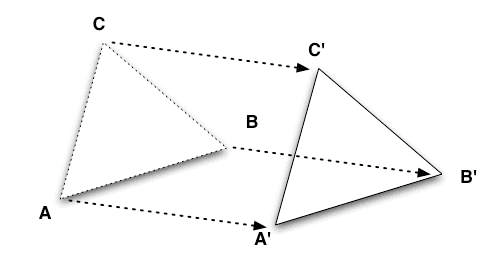
What type of transform takes ABCD to A’B’C’D’?
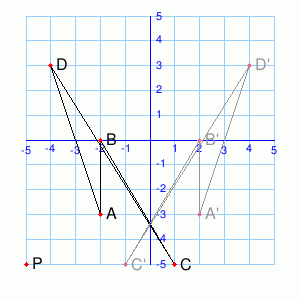
Solution
The type of transform takes ABCD to A’B’C’D’ is Reflection
Simple Problems on Combined Transformations
Solve simple problems on combined transformations
Exercise 5
What type of transform takes ABCD to A’B’C’D’?
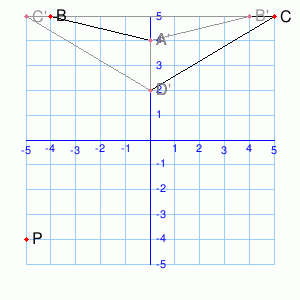
The
transformation ABCD → A’B’C’D’ is a rotation around(-1, 2)by___°.Rotate
P around(-1, 2)by the same angle. (You may need to sketch things out on
paper.)P’ = (__,__)
transformation ABCD → A’B’C’D’ is a rotation around(-1, 2)by___°.Rotate
P around(-1, 2)by the same angle. (You may need to sketch things out on
paper.)P’ = (__,__)
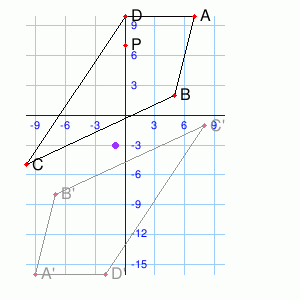
The
transformation ABCD → A’B’C’D’ is a rotation around(-1, -3)by__°Rotate P
around(-1, -3)by the same angle. (You may need to sketch things out on
paper.)P’ = (__,__)
transformation ABCD → A’B’C’D’ is a rotation around(-1, -3)by__°Rotate P
around(-1, -3)by the same angle. (You may need to sketch things out on
paper.)P’ = (__,__)
